Porosity and permeability – the flow of water and other geofluids
This is part of the How To…series on the Mineralogy of sandstones
Nearly all geological processes require the presence of water in one form or another. Most sedimentation occurs in water (aeolian deposits are the obvious exception). Sediment burial and compaction involve the expulsion of water. Diagenesis would not take place in the absence of water; hydrocarbons would not migrate to traps and minerals would not be concentrated in ore bodies. Aqueous fluids under pressure reduce cohesion and friction promoting rock deformation. Metamorphism would be painfully slow, even by geological standards if it were not for the transfer of mass in hot aqueous fluids.
All these processes require not only the presence of water, but its continual movement or flow. Below Earth’s surface, the residence and flow of aqueous fluids requires two fundamental rock-sediment properties:
– voids, commonly in the form of intergranular pores and fractures, and
– connectivity among the voids.
The first of these is referred to as porosity; the second as permeability.
There are two main types of porosity: intergranular porosity that characterizes sands, gravels and mud, and fracture porosity in hard rock. Fracture porosity forms during brittle failure of hard rock or cooling of lava flows. Fracture networks that are connected can provide pathways for fluid flow even when the host rock is impervious (e.g. granite, basalt, indurated sandstone). Highly productive aquifers are not uncommon in fractured bedrock.
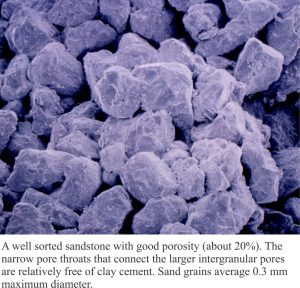
Intergranular porosity is the void space between detrital grain contacts and is expressed as a percentage of the total sediment-rock volume. It is a dimensionless number (i.e. it has no units of measure). All sediments begin life with some porosity. Well sorted beach, river and dune sands have initial porosities ranging from 30% – 40%, muds as high as 70%. These values represent the total void space, namely the large pores plus lots of microporosity in tiny nooks and crannies between grains and crystals. Hydrogeologists have found it useful to define effective porosity as that which permits easy movement of fluid. This excludes microporosity where surface tension forces inhibit flow. Effective porosity is always less than total porosity. Follow this link to a simple experiment designed to measure porosity.
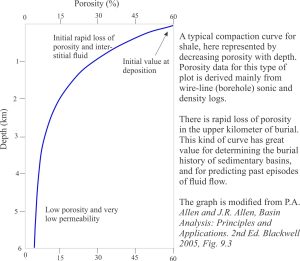
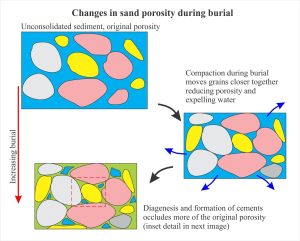
As sediment is buried, the grains settle (i.e. they become more closely packed) as they begin to compact. The reduction in porosity by mechanical compaction continues during sediment burial, in concert with the precipitation of cements (chemical diagenesis). This is particularly evident during the compaction of mud. The high initial porosity of mud is due to micro-pores between clay particles that have dimensions measured in microns. Compaction compresses the clays and drives off the interstitial water. Compaction (porosity-depth) curves for mud, like the example shown below, typically show a loss of porosity that at shallow depths is almost exponential, becoming approximately linear at depths where shale forms; total porosities in shale are extremely low.
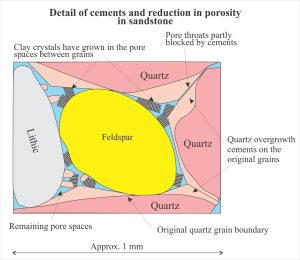
The conduits for fluid flow (water, oil, gas) from one pore space to another are the narrow connections adjacent to grain contacts. These connections are commonly referred to as pore throats. Pore throats are susceptible to blockage during sediment compaction (lithic sandstones are prone to this) and by cementation, particularly clay cements.
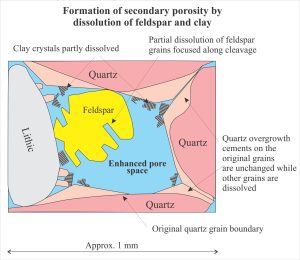
Porosity can also be enhanced during burial diagenesis. The primary mechanism for formation of secondary porosity is the dissolution, or partial dissolution of framework grains like feldspar and carbonate bioclasts. Many of these secondary pores are larger than the associated intergranular pore spaces; this is an important diagnostic clue to their identification. Likewise, carbonate and clay cements may be prone to dissolution, resulting in enhanced post-depositional porosity.
Burial depths and temperatures where formation of secondary porosity is encountered commonly coincide with chemical reactions involving the break-down of organic matter. By-products of these reactions include carbon dioxide (and carbonic acid) and organic acids like acetic acid. There is a fundamental shift in pH and chemical equilibria, particularly for carbonates, and this promotes dissolution.
Secondary porosity can also form during subaerial exposure of rock and by bioturbation. However, the secondary porosity seen in most ancient sandstones is the product of burial diagenesis.
Permeability measures the ease with which a fluid flows through sediment or rock. The flow of fluid from one part of a rock to another, or from an aquifer to a bore hole, depends on the connections among pores and fractures. It is possible for a rock or sediment to have high porosity but low permeability if the intergranular or intercrystal connectivity is low – mud and shale are prime examples. In coarse-grained sediments that are devoid of clay, there is a good correlation between porosity and permeability. This relationship does not apply where there are significant amounts of clay.
Permeability can be expressed in two ways. Henry Darcy’s pivotal experiments with sand-filled tubes (in 1856) established an empirical relationship between hydraulic gradient (that basically is an expression of hydraulic potential energy) and discharge. The proportionality constant in this relationship is called the hydraulic conductivity (K) (a label borrowed from electrical theory), that has units of distance and time (cm/s, feet/s). In mathematical terms, hydraulic conductivity is expressed as a velocity, also known as the Darcy velocity. Hydraulic conductivity is the standard expression of permeability in groundwater studies. Its value depends not only on the connectivity of pores but also on the dynamic viscosity and density of the fluid (viscosity measures the resistance to flow – crude oil is more viscous than water). Thus, for any porous medium the value of K will be different for water and oil, a factor that is important in groundwater remediation.
The hydrocarbon industry deals with fluids of highly variable viscosity (water, oil, gas) and has opted for a standard expression of intrinsic permeability (k) that depends only on the porous medium. The unit is the Darcy that mathematically reduces to units of area (ft2, m2). It is basically a measure of pore size (the oil industry commonly uses the term millidarcy). Frequently used conversions to Darcys are:
1 m2 = 1.013 x 1012 Darcy
1 Darcy = 9.87 x 10-13 m2
Hydraulic conductivity (K) and intrinsic permeability (k) are related by fluid density and dynamic viscosity such that:
k (m2) = K (m/s) x (1.023 x 10-7 m.s) (the time components cancel)
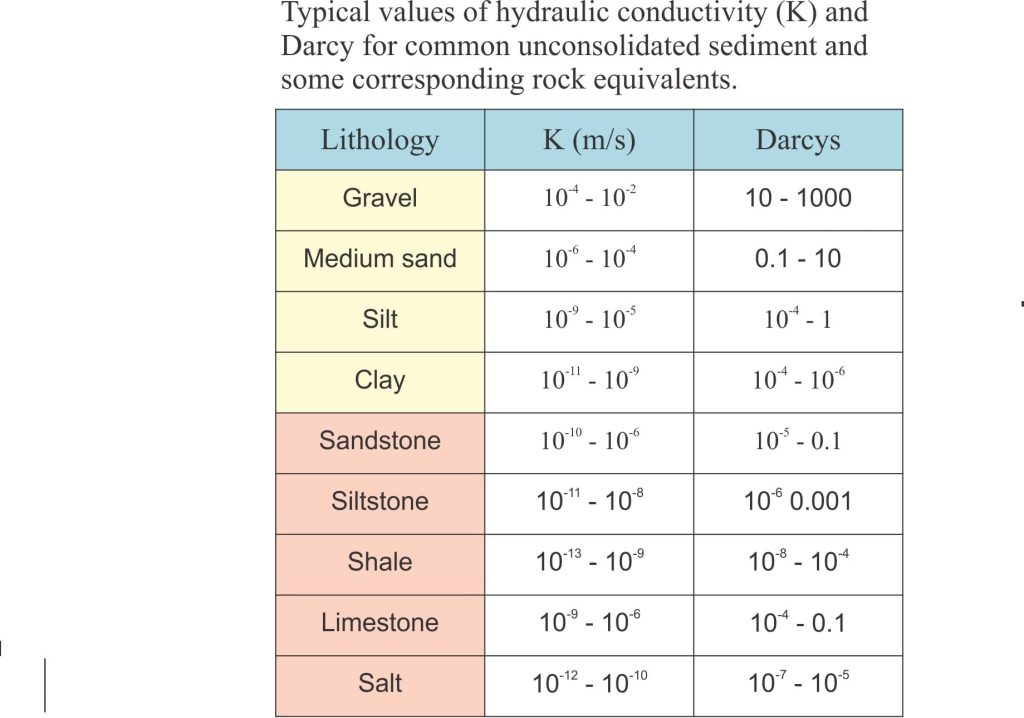
Typical permeability values for unconsolidated sediment and some rock equivalents are shown in the table below.
As you can see, the permeability of shale is extremely low. This is the reason why shale beds make good seals to hydrocarbon reservoirs, and aquitards to confined aquifers. Fluid flow in shales and well-cemented sandstones or limestones can be enhanced by hydraulic fracturing. This process (fracing) is front and centre of shale oil production (notwithstanding all the pros and cons of this industrial process). But that is a story for another time.
Here are three excellent texts that detail the theoretical aspects of the above:
P.A. Allen and J.R. Allen, Basin Analysis: Principles and Applications. Blackwell 2005
C.W. Fetter. Applied Hydrogeology, 2001. PrenticeHall
P.A.Domenico and F.W.Schwartz Physical and Chemical Hydrogeology,1998 John Wiley & Sons